Finding the Time complexity (Big "O") of programs meant to obtain the initial coefficients of a binomial expansion in MQL4.

[Chinedu Peter]

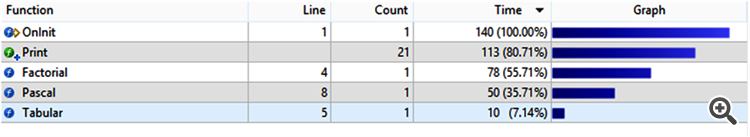
The following three blocks of codes are meant to find the initial coefficients of the expansion of a binomial expression up to power 6. That is, since (x + y)^6 = x^6 + 6x^5y + 15x^4y^2 + 20x^3y^3 + 15x^2y^4 + 6xy^5 + y^6, the program is meant to obtain the numbers 1, 6, 15, 20, 15, 6, 1 given only the input 6. Each block of code represents one of three different methods of finding the numbers. The question is: What are the Time complexity (Big "O") of each block of codes given that the input is 6 in each method? Thank you as I anticipate your answers.

First method - THE USE OF PASCAL'S TRIANGLE

void OnInit()
  {
   int P0, P1, P2, P3, P4, P5, P6, P7, P8,P9, P10, P11, P12, P13, P14;
   int I1, I2, I3, I4, I5, I6, I7, I8,I9, I10, I11, I12, I13;
   for (int row = 0; row <= 6; row++)
     {
      for (int col = 1; col <= 13; col++)
        {
         if (row == 0)
           {
            I7 = 1;
           }
         else 
           {
            I1 = P0 + P2;
            I2 = P1 + P3;
            I3 = P2 + P4;
            I4 = P3 + P5;
            I5 = P4 + P6;
            I6 = P5 + P7;
            I7 = P6 + P8;
            I8 = P7 + P9;
            I9 = P8 + P10;
            I10 = P9 + P11;
            I11 = P10 + P12;
            I12 = P11 + P13;
            I13 = P12 + P14;
           } 
        }
      P1 = I1; P2 = I2; P3 = I3; P4 = I4; P5 = I5; P6 = I6; P7 = I7; P8 = I8; P9 = I9; P10 = I10; P11 = I11; P12 = I12; P13 = I13;
     }
   if(I1 != 0) Print(I1);
   if(I2 != 0) Print(I2);
   if(I3 != 0) Print(I3);
   if(I4 != 0) Print(I4);
   if(I5 != 0) Print(I5);
   if(I6 != 0) Print(I6);
   if(I7 != 0) Print(I7);
   if(I8 != 0) Print(I8);
   if(I9 != 0) Print(I9);
   if(I10 != 0) Print(I10);
   if(I11 != 0) Print(I11);
   if(I12 != 0) Print(I12);
   if(I13 != 0) Print(I13);
  }

Second method - THE USE OF FACTORIAL

void OnInit()
  {
   int I1, I2, I3, I4, I5, I6, I7;
   I1 = (6 * 5 * 4 * 3 * 2 * 1) / ((1) * (6 * 5 * 4 * 3 * 2 * 1));
   I2 = (6 * 5 * 4 * 3 * 2 * 1) / ((1) * (5 * 4 * 3 * 2 * 1));
   I3 = (6 * 5 * 4 * 3 * 2 * 1) / ((2 * 1) * (4 * 3 * 2 * 1));
   I4 = (6 * 5 * 4 * 3 * 2 * 1) / ((3 * 2 * 1) * (3 * 2 * 1));
   I5 = (6 * 5 * 4 * 3 * 2 * 1) / ((4 * 3 * 2 * 1) * (2 * 1));
   I6 = (6 * 5 * 4 * 3 * 2 * 1) / ((5 * 4 * 3 * 2 * 1) * (1));
   I7 = (6 * 5 * 4 * 3 * 2 * 1) / ((6 * 5 * 4 * 3 * 2 * 1) * (1));
   Print(I1);
   Print(I2);
   Print(I3);
   Print(I4);
   Print(I5);
   Print(I6);
   Print(I7);
  }

Third method: THE TABULAR METHOD

void OnInit()
  {
   int I1, I2, I3, I4, I5, I6, I7;
   I1 = 1;
   I2 = (6 * I1) / 1;
   I3 = (5 * I2) / 2;
   I4 = (4 * I3) / 3;
   I5 = (3 * I4) / 4;
   I6 = (2 * I5) / 5;
   I7 = (1 * I6) / 6;
   Print(I1);
   Print(I2);
   Print(I3);
   Print(I4);
   Print(I5);
   Print(I6);
   Print(I7);
  }

[William Roeder]

macpee: What are the Time complexity (Big "O") of each block of codes g

Just run it through the profiler

[Chinedu Peter]

William Roeder #:

Just run it through the profiler

Thanks bro.

[Chinedu Peter]

William Roeder #:

Just run it through the profiler

I combined the three methods in one program and named the codes accordingly and the above is the result of the profiling. What now is the Time complexity, is it n or n-square or log n or exp(n)?