Impulse - page 7

[Renat Akhtyamov]

Олег avtomat:
I don't understand... what's on the parabola?

How would it all look if a parabola was inserted instead of a function?

[[Deleted]]

add a bit of fast quadratic growth (get a parabola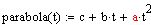 with superimposed oscillations)

[[Deleted]]

new-rena:
What would it all look like if you replaced the function with a parabola?

Well, a parabola is also a function.

[Vladimir Karputov]

Олег avtomat:

add a little quick quadratic growth

Interesting correlations. And the change in forex price can be roughly described by which equation from the ones you gave?

[[Deleted]]

Karputov Vladimir:
Interesting correlations. But the change in forex price can be roughly described by which equation of the ones you cited?

I clarified there, meaning a parabola

[Renat Akhtyamov]

Олег avtomat:

I clarified there, meaning the parabola

I see. simpler: s(t)=parabola, what do you get?

[[Deleted]]

new-rena:
I see. simpler: s(t)=parabola, what do you get?

A parabola is a line of second order. Its acceleration is a constant, so the derivative of the acceleration (jerk) is zero :

[Renat Akhtyamov]

Олег avtomat:

A parabola is a line of second order. Its acceleration is a constant, so the derivative of the acceleration (jerk) is zero :

ah, well, yes. i.e. it is possible that at jerk=0 there is a beginning/end of momentum?

[[Deleted]]

Karputov Vladimir:
Interesting correlations. But the change in forex price can be approximated by which of the equations you cited?

If we do not impose any proximity requirements (like MOC, etc.), this formulation is incorrect.

The same interval of price movement can be described by a straight line, or by a sinusoid, or by a complex set of different components. Everything will depend on the purposes of such description, and these purposes dictate an acceptable degree of approximation closeness to the original series.

[[Deleted]]

new-rena:
ah, right. i.e. is it possible that at jerk=0 there is a start/end impulse?

Real motion will never be a pure parabola, even assuming no superimposed oscillations, as there is always noise present that chatter not only the third derivative (i.e. jerk != 0 ), but also derivatives of higher orders.